Raul Fattore
May 27, 2024
This study is divided into four parts
Table of Contents
Summary of Part 2
The Universal Force provides a superior method for calculating total energy for a system of charges, including kinetic variables like velocity and acceleration. This formula is absent in Relativity Theory and other theories. The relationship between force and total energy is demonstrated for zero-force, zero-energy, maximum force, and maximum energy. The interaction distance between single charges, nuclei, atoms, or molecules is dynamic and depends on system kinetics, contradicting the “Van Der Waals radii” assigned to elements in the periodic table.
Abstract
The increasing need for energy by mankind over time has led to climate change problems and, most importantly, human health issues due to the storage of radioactive waste that lasts a very long time, thus affecting future generations.
Advances in atomic and nuclear physics several years ago promised a revolution in energy production, offering a significant advantage over burning coal, gas, or oil. Unfortunately, scientists at that time did not make use of their brains appropriately. They simply misused and wasted all the advanced knowledge acquired in atomic and nuclear physics to just boil water!
Nuclear fusion was “reborn” as an alternative to generate energy that might be “clean” for the environment, with “minimum” radiation risks for mankind and no radioactive waste. Clean energy generation and radiation-free risks can only be guaranteed by using certain technology for nuclear fusion.
Making a fusion reaction happen is not complicated (like 14-year-old Taylor Wilson did in his home in 2008). However, there are two main problems with nuclear fusion that have prevented it from being commercially viable until now:
- Efficiency
- Safety
Currently, most scientists experimenting with nuclear fusion are making serious mistakes. They are using costly devices and wasting vast amounts of energy in an attempt to replicate conditions found in stars on Earth, with no encouraging outcomes to date. Why? Fusions in stars are processes that are fully determined by probability, so you cannot build a project just on them. Probability is not the same as certainty, even when it represents the likelihood that an event will occur.
Negative mass calculations can provide certainty regarding an effective head-on collision of charges, but scattering cross-section calculations cannot.
Nuclear fusion can be greatly eased by negative mass. I refer the reader to the series of articles about Negative Mass in Atom Nuclei [1] to understand how we can have negative mass behavior in nuclei.
- Is nuclear fusion enhanced with negative mass nuclei and particles?
- Can negative mass nuclear fusion be made efficient?
- Can negative mass nuclear fusion offer better safety?
- Does negative mass nuclear fusion rely on statistical processes?
- Does negative mass nuclear fusion offer a simpler and cheaper method to generate energy?
In the development of this study, you’ll find comprehensible answers to the above questions, which are backed by the experimentally proven laws of electrodynamics through the Universal Electrodynamic Force and the New Atomic Model.
Introduction
Nuclear fusion is an extremely fast and violent event that involves the acceleration of charges, whether they are nuclei or single charges. Therefore, scientists should not lose sight of the fact that in any atomic and nuclear interaction, we are dealing with accelerated electric charges.
As such, radiation is always present in any nuclear fusion reaction. No exception.
Due to the huge acceleration of the charges, the spectrum may easily range from X-rays to the most powerful gamma radiation. This radiation can be used in our favor to generate electric energy [9]. However, if this radiation is not properly handled, it will be harmful for human health.
For simplicity, the radiation symbols will be omitted in most of the reaction calculations throughout this study. However, as radiation is always present in any nuclear fusion, from X-ray to gamma, the right way to write a reaction should be to include the radiation symbols as part of the fusion products: A+B\ \rightarrow\ C+D\ +\ \left(X,\ \gamma\right).
Unfortunately, scientists are making deficient calculations of the released energy during nuclear fusion. Why?
Because they use wrong formulas that don’t give the total energy of the system, like the known Einstein’s formula E=\Delta m\ c^2 for mass excess/defect calculations. This formula only gives a result for rest mass energy (potential energy), which should be equivalent to the acquired kinetic energy, but it gives no clues about acceleration. The Einstein energy formula is flawed even in its “relativistic” form since, as in classical mechanics, acceleration is entirely disregarded.
The total energy in a fusion reaction is always higher than the results given by Einstein’s formulas for rest energy and the calculations of kinetic energy from classical mechanics.
Most of the energy values for nuclear fusion given in the scientific literature are deficient because none of them consider radiation energy, which on occasion may be much higher than kinetic energy alone.
Applying Einstein’s formulas and classical mechanics’ kinetic energy calculations to nuclear fusion is extremely risky, since you can never be certain of the amount of radiation generated during a reaction.
The scientific community must be aware when using those formulas because:
• They only give partial energy values.
• They are not compatible with a system of charges.
Those formulas may only be applied to limited, simple mechanical interactions between uncharged bodies.
The derivation of the total energy for a system of charges made in this study will provide scientists with a powerful formula to make real energy calculations in any nuclear fusion reaction and many other applications that involve the interaction of charges.
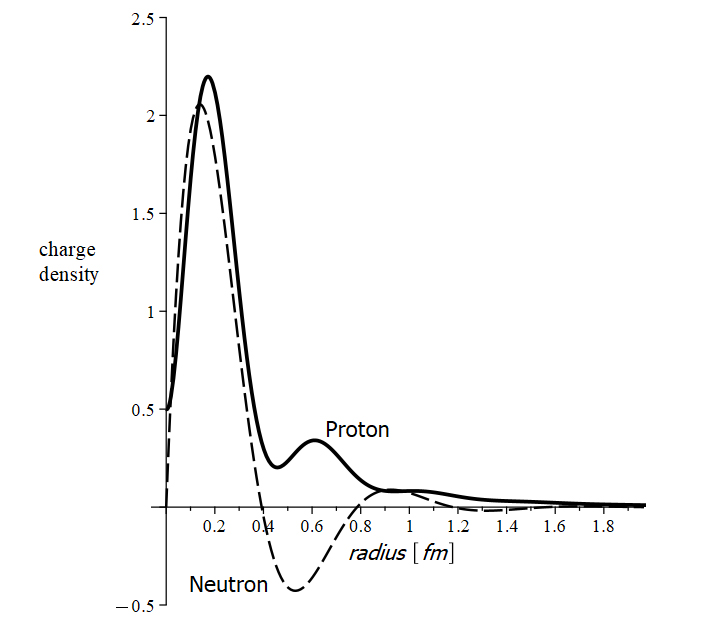
Charge density of the proton and neutron
There are no “neutral particles”. The so-called “neutral particles” are composed of charges that balance the net result [2]. An example of such a particle is the neutron.
Neutrons are stable in the nucleus. However, outside the nucleus, the neutron decays into a proton and an electron with a half-life of fewer than 15 minutes. The mass of the neutron is the sum of the masses of the electron and proton. The neutron has a charge density that varies between positive and negative with respect to its radius (Fig. 1). These facts suggest that the neutron might not be a valid elementary particle but a bound combination of an electron and a proton [3].
Accordingly, the new atomic model precisely describes how electrons and protons are very tightly packed in shells in the nucleus due to the balance of electromagnetic forces.
Based on the energy calculations I made for the Deuterium-Tritium fusion, the binding energy (potential energy) of the electron-proton pair that makes the neutron should be E=-2.127\ MeV when in the nucleus. Outside the nucleus, this energy is given off (radiation, kinetic) during neutron decay time, which unbinds the electron-proton pair.
Because the neutron is always regarded as a “neutral” particle, scientists’ calculations of nuclear reactions and stray reactions may only be partially accurate. The electron and proton may take part in reactions with nuclei or with other electrons and protons when the electron-proton pair that built the neutron unbinds. While some of these reactions will produce energy, others may use some of the system’s energy to generate the fuel for fusion.
As far as I know, these “additional” reactions were never mentioned in any scientific paper. However, some of them could be of such importance that they cannot be ignored and must be considered in calculations, as will be demonstrated in the development of this study.
Since the neutron is one of the fusion products in several reactions and usually carries a high level of kinetic energy and radiation energy, we might be able to use these facts and the internal structure of the neutron in our favor.
The fact that the neutron is a composite of two charges means that we may expect a radiation spectrum from X-rays to gamma rays when the particle is in positive or negative accelerated motion. This is additional energy that we may harvest from the “neutron”, which can be converted into electricity, besides the charges themselves.
Total Energy of a System of Charges Derived from the Universal Force
Before we derive the general equation for the total energy of a system of charges, let’s see how to calculate the necessary energy to bind and unbind a system of charges.
Total Energy to Bind a System of Charges
The total energy needed to bind a system of charges (called “potential energy”) is defined as the total energy change (or work done) caused by the force between two different states of the system:
E=\int_{r_i}^{r_f}{-{\vec{F}}_u}d\vec{r} (1)
Where E is the total energy of the system, r_i and r_f are the initial and final distance between the charge centers.
The initial distance in the integral of the force is usually taken such that the rest energy of the system is approximately zero, which usually means r=\infty. But as infinity is not a defined number, let’s take a practical value from Mother Nature that can realistically be used instead. Infinity can be replaced by a huge distance, approx. x10 bigger than the oldest light we have observed from the “Big Bang” ({46500\ 10}^6ly={4.4\ 10}^{26}m). To go safe, let’s take r_i={10}^{27}m.
The final distance is the average distance between shells of charge for the given binding energy, which is the energy we must supply to break a nucleus or a bound of charges.
Total Energy to Unbind a System of Charges
The total energy needed to unbind a system of charges is equal to and opposite to the binding energy. In this case, we must switch the integral limits to calculate the total energy, as the process is the opposite of the one above. The total energy we need to supply to the system is defined as the total energy change (or work done) caused by the force between two different states of the system:
E=\int_{r_f}^{r_i}{-{\vec{F}}_u}d\vec{r} (2)
Total Energy of a System of Charges Valid for Any Type of Motion
As stated in Part 1, the laws of electrodynamics are valid on any scale.
There is no reason for the laws of electrodynamics not to be valid at atomic scales. There is no such thing as “Nuclear Strong Force” or “Nuclear Weak Force” as proposed by Quantum Theory. There is only one force in Mother Nature that is valid on any scale: the Universal Electrodynamic Force. It can clearly explain atomic and nuclear interactions without any need to create any new forces, as Quantum Theory did.
The Total Energy of moving charges is derived from the Universal Electrodynamic Force, which gives us a more complete and accurate energy equation. The derived Total Energy equation has three terms:
1) The Potential Energy term, which depends on the relative position of the charges.
2) One Kinetic Energy term, which depends on the relative velocity of the charges.
3) One Kinetic Energy term, which depends on the relative acceleration of the charges. This term accounts for radiation energy and is not found in the scientific literature as being part of the total energy of the system.
Moreover, the three terms of the total energy are multiplied by a factor that depends on the type of motion and velocity. This factor is analogous to the “Lorentz factor” but is physically realistic because it is valid for any type of motion, while the “Lorentz factor” is only valid for circular motion. This demonstrates a big flaw in the Theory of Relativity.
The Universal Electrodynamic Force [1] for any motion is given in geometrical form by the following formula:
F_u=\frac{k\cdot q_1\cdot q_2}{r^2}\cdot\frac{\left(\left(1-\beta^2\right)\cdot r+\frac{2\cdot r^2}{c^2}\cdot a\cdot\cos{\left(\alpha\right)}\right)}{r\cdot\left(1-\beta^2\cdot\sin^2{\left(\theta\right)}\right)^\frac{1}{2}}-\frac{k\cdot q_1\cdot q_2}{r^2}\cdot\frac{\left(1-\beta^2\right)\cdot\left(\left(\beta^2\cdot r^2\cdot\cos^2{\left(\theta\right)}+\frac{r^3\cdot a\cdot\cos{\left(\alpha\right)}}{c^2}\right)\cdot r-\beta\cdot r^3\cdot\cos{\left(\theta\right)}\cdot\frac{v\cdot\cos{\left(\theta\right)}}{c}-\frac{r^4}{c^2}\cdot a\cdot\cos{\left(\alpha\right)}\right)}{r^3\cdot\left(1-\beta^2\cdot\sin^2{\left(\theta\right)}\right)^\frac{3}{2}} (3)
Where \beta=\frac{v}{c}, and r,\ v,\ a are the relative distance, velocity, and acceleration between the charges, while \theta is the angle between \vec{r} and \vec{v}, and \alpha is the angle between \vec{r} and \vec{a}.
As stated in previous paragraphs, the total energy of the system is defined as the total energy change (or work done) caused by the force between two different states of the system. So, let’s integrate the Universal Force (3):
E=\int_{r_i}^{r_f}{-{\vec{F}}_u}d\vec{r}The result of the integral after doing some algebra gives us the total energy for a system of two charges:
E=\frac{1}{\sqrt{1+\left(\cos^2{\left(\theta\right)}-1\right)\frac{v^2}{c^2}}}\cdot\left(kq_1q_2\cdot\left(\frac{1}{r_f}-\frac{1}{r_i}\right)-\frac{kq_1q_2}{c^2}\cdot\left(\frac{1}{r_f}-\frac{1}{r_i}\right)\cdot v^2-\frac{2kq_1q_2\cos{\left(\alpha\right)}}{c^2}\cdot\left(\ln{\left(r_i\right)}-\ln{\left(r_f\right)}\right)\cdot a\right) (4)
As we can see, the total energy is affected by a factor \gamma_E=\frac{1}{\sqrt{1+\left(\cos^2{\left(\theta\right)}-1\right)\frac{v^2}{c^2}}} that is physically realistic compared to the known “Lorentz factor”, because it also takes into account the type of motion that is given by the angle \theta between \vec{r} and \vec{v} (the relative position and velocity). Note that the “Lorentz factor” is only valid for circular motion. We see an important mistake in the Theory of Relativity, which is unable to describe the variables for any type of motion.
We can write the total energy as follows:
E=\gamma_E\cdot\left(kq_1q_2\cdot\left(\frac{1}{r_f}-\frac{1}{r_i}\right)-\frac{kq_1q_2}{c^2}\cdot\left(\frac{1}{r_f}-\frac{1}{r_i}\right)\cdot v^2-\frac{2kq_1q_2\cos{\left(\alpha\right)}}{c^2}\cdot\left(\ln{\left(r_i\right)}-\ln{\left(r_f\right)}\right)\cdot a\right) (5)
Where 1\le\gamma_E\le\infty depending on the angle \theta between \vec{r} and \vec{v} and the relative velocity, reaching a maximum value (or \infty) for \theta=\frac{\pi}{2}.
The total energy in the Theory of Relativity is incomplete because it doesn’t include an acceleration term, as we have derived here from the Universal Electrodynamic Force. In the Theory of Relativity, the only kinetic variable is velocity. But you will never know what acceleration was needed to reach that velocity. This is a serious error in that theory.
As \gamma_E affects the whole equation, let’s analyze only the terms inside the parentheses to find the meaning of each one of them.
The first term is the equivalent to the potential energy “U” due to the electrostatic fields, U=kq_1q_2\cdot\left(\frac{1}{r_f}-\frac{1}{r_i}\right). The other two terms are kinetics.
The second term is equivalent to the kinetic energy “K”, which we can write as K=-\frac{kq_1q_2}{c^2}\left(\frac{1}{r_f}-\frac{1}{r_i}\right)\cdot v^2=-\frac{1}{2}\cdot m_{sys}\cdot v^2, with m_{sys}=\frac{2kq_1q_2}{c^2}\left(\frac{1}{r_f}-\frac{1}{r_i}\right) being the equivalent mass of the system.
The third term involving acceleration is also a kinetic energy term responsible for radiation energy. The Universal Electrodynamic Force contains a term that causes the helical motion (corkscrew motion) of charges. This term is responsible for radiation. The acceleration here is the centripetal acceleration, so we can make a mechanical equivalent of the rotational kinetic energy: K_{rot}=\frac{1}{2}I\omega^2=\frac{1}{2}mr^2\omega^2=\frac{1}{2}mr\cdot\left(r\omega^2\right)=\frac{1}{2}mra, being \frac{1}{2}mr=\frac{2kq_1q_2}{c^2}\cdot\left(\ln{\left(r_f\right)}-\ln{\left(r_i\right)}\right).
But in our system of charges, it makes more sense to define this term as the radiation energy of the system E_{rad}. Therefore, we can write the total energy of the system in short form as:
E=\gamma_E\left(U+K+E_{rad}\right) (6)
The “rest energy” is no other than the potential energy “U” when the kinetic variables are zero (v=0 and a=0). The Universal Force shows us that velocity zero doesn’t mean that acceleration should be zero. Note that in this case \gamma_E=1. Under such conditions, the rest energy is:
E=E_0=URecall that the “rest energy” depends on the distance between the centers of charges. In the nucleus, it is given by the average distance among the shells of charge.
The Total Energy Under Head-On Collision Conditions in Proton-Proton Fusion
Let’s analyze the case of the fusion of two protons that we introduced in Part 1. For this motion condition \gamma_E=1, and making r_i={10}^{27}m, r_f=r, the total energy (Eq.5) can be re-written as follows:
E=k\cdot q_1\cdot q_2\cdot\left(1-\frac{v^2}{c^2}\right)\cdot\left(\frac{1}{r}-{10}^{-27}\right)+\frac{k\cdot q_1\cdot q_2}{c^2}\cdot\left(2\cdot\ln{\left(r\right)}-124.34\right)\cdot a (7)
According to mass defect (excess) calculations, the fusion of two protons should generate Q=1.44\ MeV.
Let’s do the calculation by using Eq. (7), based on the average radius of the proton of r=0.85\ {10}^{-15}m.
E=2.707576470\times{10}^{-13}-3.008418300\times{10}^{-30}v^2-4.954299420\times{10}^{-43}a\ \ [Joules]By assuming low relative velocity and acceleration, we may disregard the kinetic terms. In this case, we see that the energy of the fusion’s products is E_0=1.7\ MeV.
The same calculations done for the experimentally obtained radius of the proton, r=0.8783\ {10}^{-15}m, returns the following result:
E=2.620334737\times{10}^{-13}-2.911483041\times{10}^{-30}v^2-4.952624388\times{10}^{-43}a\ \ [Joules]By assuming low relative velocity and acceleration, we may disregard the kinetic terms. The energy is E_0=1.63\ MeV.
The New Atomic Model [1] predicts a high elasticity of the charges, which yields a drastic change in size according to their organization, kinetics, etc. Therefore, both the estimated and experimentally measured radius should never be taken as a “fixed and unique” dimension for any condition.
Assuming that during the fusion the protons “adopt” a radius of r=1\ {10}^{-15}m, we obtain the following total energy:
E=2.301440000\times{10}^{-13}-2.557155556\times{10}^{-30}v^2-4.945987698\times{10}^{-43}a\ \ [Joules]Again, by assuming low relative velocity and acceleration, we may disregard the kinetic terms. Now, we see that the energy is E_0\cong1.44\ MeV, which exactly matches the value calculated with the mass defect (excess).
This constitutes another proof that the Laws of Electrodynamics are valid on any scale and can safely be used to obtain more comprehensive results in all cases.
Remark: The energy results above were quickly obtained based on the rest energy (or potential energy) by dismissing the kinetics of the system. Even when the potential energy is equivalent to the kinetics of the system, this calculation procedure gives deficient outcomes and should be avoided because no result of radiation energy is obtained. In Part 3, it is explained in detail how to calculate the real released energy and the single energy of the products.
The graph of the total energy vs. velocity and acceleration (Fig. 2) for r={1\ 10}^{-15}m, shows us how the energy changes in magnitude and sign according to the kinetics of the charges:
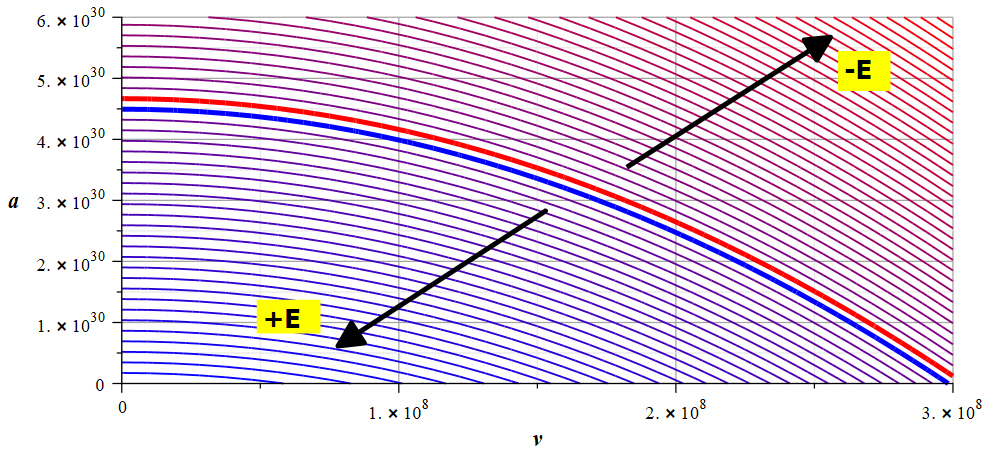
Total Energy in the fusion of two protons for a certain distance between charge centers
The thick blue and red lines delimit the region of zero energy. The energy of the two protons at some distances is negative for certain combinations of relative velocity and acceleration. Note that for charges of the opposite sign, the sign of the energy is reversed in the graph.
How does the total energy change with the relative distance under such conditions?
The graph in Fig. 3 shows the total energy vs. distance for the following values of relative velocity and acceleration: v=10\frac{m}{s} and a=3\ {10}^{30}\frac{m}{s^2}.
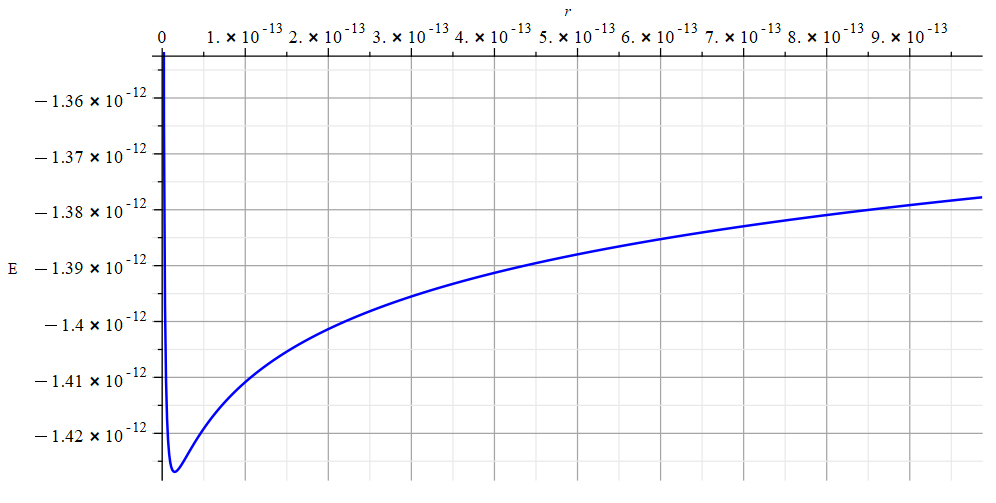
Total Energy vs. distance in the fusion of two protons
The zero-energy points can be calculated by setting Eq. (7) to zero:
E=k\cdot q_1\cdot q_2\cdot\left(1-\frac{v^2}{c^2}\right)\cdot\left(\frac{1}{r}-{10}^{-27}\right)+\frac{k\cdot q_1\cdot q_2}{c^2}\cdot\left(2\cdot\ln{\left(r\right)}-124.34\right)\cdot a=0 (7a)
The analytical solutions to this equation in the variable “r” involve the Lambert function: W\left(x\right)\cdot\mathrm{e}^{W\left(x\right)}=x
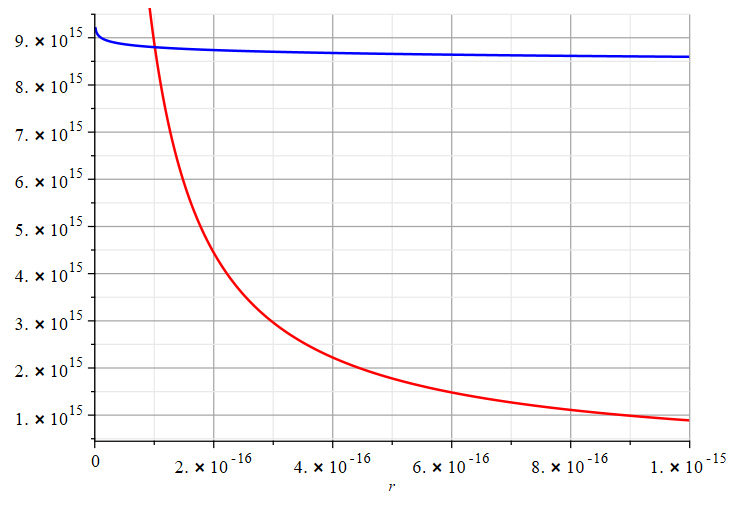
Graph of the two functions
One easy way to find the solutions is to graph the functions in both terms and find the coordinate “r” of the intersection points, for some given values of “v” and “a”:
\left(1-\frac{v^2}{c^2}\right)\cdot\left(\frac{1}{r}-{10}^{-27}\right)=-\frac{1}{c^2}\cdot\left(2\cdot\ln{\left(r\right)}-124.34\right)\cdot aFrom the graph of these two functions (Fig. 4) for v={10}^8\frac{m}{s} and a=4\ {10}^{30}\frac{m}{s^2}, we see that the intersection point is at r=1\ {10}^{-16}m.
Another easy way is to solve Eq. (7a) for “r” for the values of velocity and acceleration given above. We’ll get two solutions for the system: r_1={10}^{27}m and r_2={1.01\ 10}^{-16}m. The first solution is the largest distance for zero-energy (~ \infty), while the second solution is the smallest value we were interested in finding.
The analytical solutions by applying the Lambert function take some algebraic work. However, we also get the two solutions for the system: r_1={10}^{27}m and r_2={1.01\ 10}^{-16}m. The first solution is the largest distance for zero-energy (~ \infty), while the second solution is the smallest value we were seeking.
On the other hand, the minimum of the function or the maximum negative energy point can be found by taking the derivative of the energy equal to zero \frac{d}{dr}\left(E\right)=0, which gives:
{r_E}_{max}=\frac{c^2\left(1-\frac{v^2}{c^2}\right)}{2a} (8)
As the total energy is E=\int_{r_i}^{r_f}{-\vec{F}}\ d\vec{r}, then \frac{d}{dr}\left(E\right)=F=0. It means that the point of maximum negative energy coincides with the zero-force point.
The graph of Eq. (8) in Fig. 5 shows how the distance of maximum negative energy changes with the kinematic variables.
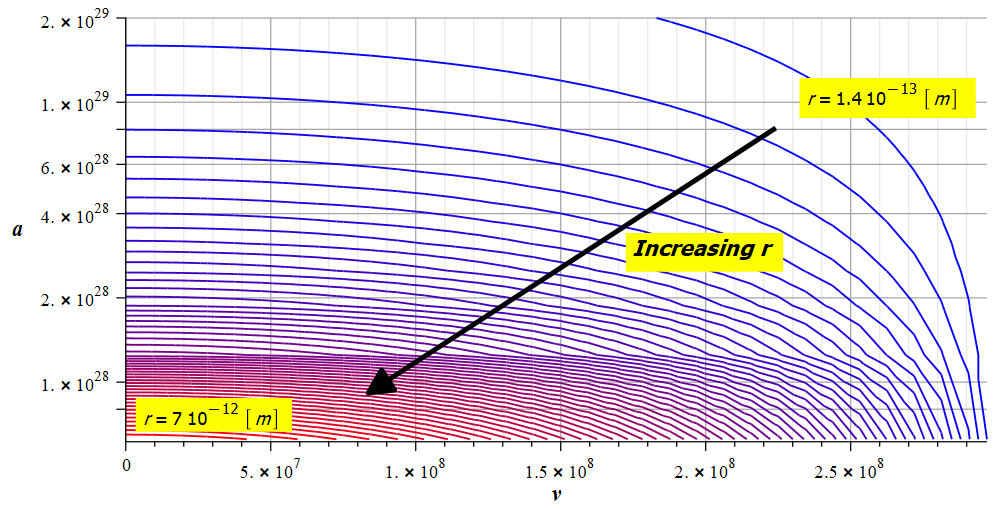
How the distance of maximum negative energy changes with the kinematic variables
Combined Graphs of Force and Total Energy
Taking the force and energy equations obtained for the fusion of two protons, with one at rest and the other brought from “infinity” in the head-on collision condition (\theta=\alpha=\pi), let’s visualize the zero points and minima of force and energy for some kinetic values. Note that the points of minima can be interpreted as the maximum negative value of the force and total energy.
The Universal Force between the two protons is given by the following equation:
F_u=\left(\frac{kq_1q_2\left(\left(1-\frac{v^2}{c^2}\right)r-\frac{2r^2a}{c^2}\right)}{r^3}-\frac{kq_1q_2\left(1-\frac{v^2}{c^2}\right)\left(\left(\frac{v^2r^2}{c^2}-\frac{r^3a}{c^2}\right)r-\frac{v^2r^3}{c^2}+\frac{r^4a}{c^2}\right)}{r^5}\right) (9)
While the total energy for the system of two protons is:
E=k\cdot q_1\cdot q_2\cdot\left(\left(1-\frac{v^2}{c^2}\right)\cdot\left(\frac{1}{r}-{10}^{-27}\right)+\frac{1}{c^2}\cdot\left(2\cdot\ln{\left(r\right)}-124.34\right)\cdot a\right) (10)
Figure 6 shows the graph of the force and total energy for the system of two protons for the following parameters: v={10}^8\frac{m}{s} and a=4\ {10}^{30}\frac{m}{s^2}.
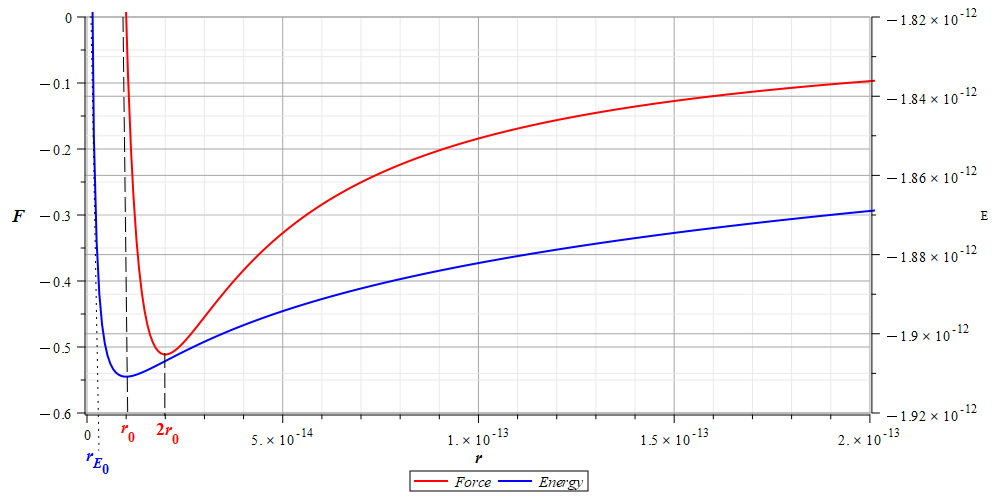
Force and total energy in a system of two protons under a head-on collision condition
The distance of zero-energy {r_E}_{0} is calculated from the energy equation equated to zero and solving for {r_E}_{0} (Lambert function solution). Note that, because of scaling, the zero-energy value is not shown in Fig. 6.
The distance of zero-force is the point of maximum negative energy (minimum of energy function):
r_0={r_E}_{max}=\frac{c^2\cdot\left(1-\frac{v^2}{c^2}\right)}{2a}The distance of “minimum” force (maximum negative force) is given by:
2r_0={r_F}_{max}=\frac{c^2\cdot\left(1-\frac{v^2}{c^2}\right)}{a}We see that for a given system of two charges, the relative distances between the centers of charges for energy zero ({r_E}_0), maximum energy (r_0), zero-force (r_0) and maximum force (2r_0), are not specific values of a system of charges with certain radii. Those relative distances depend on the kinetics of the interacting charges, such as their relative velocity and acceleration.
The Lennard-Jones Potential model as well as the Van Der Waals interactions, Van Der Waals force, and Van Der Waals radius are not based on the laws of Electrodynamics.
The interaction distance between single charges, nuclei, atoms, or molecules is dynamic and depends on the kinetics of the system. Thus, assigning fixed values of “Van Der Waals radii” to the elements in the periodic table is completely wrong.
The Total Energy Under Head-On Collision Conditions in Electron-Electron Fusion
The same procedure used in the proton-proton fusion from the previous section applies, so you can refer to the graphs and calculations made there. Identical relative distances between the centers of charges for energy zero ({r_E}_0), maximum energy (r_0), zero-force (r_0) and maximum force (2r_0), apply to the fusion of two electrons.
That said, only the calculation of the total energy of the fusion will be made below.
Let’s calculate the total energy of the fusion of two electrons under a head-on collision condition. For this motion condition \gamma_E=1, and making r_i={10}^{27}m, r_f=r, the total energy (Eq. 5) can be re-written as follows:
E=k\cdot q_1\cdot q_2\cdot\left(1-\frac{v^2}{c^2}\right)\cdot\left(\frac{1}{r}-{10}^{-27}\right)+\frac{k\cdot q_1\cdot q_2}{c^2}\cdot\left(2\cdot\ln{\left(r\right)}-124.34\right)\cdot aAccording to NIST, the electron radius is r=2.8\ {10}^{-15}m. After plugging in the values, we obtain the total energy of the fusion of two electrons:
E=8.219428570\times{10}^{-14}-9.132698411\times{10}^{-31}v^2-4.893329758\times{10}^{-43}aBy assuming low relative velocity and acceleration, we may disregard the kinetic terms. In this case, we see that the rest energy (or potential energy) of the fusion product is:
E=8.219428570\times{10}^{-14}Joules=0.513\ MeV (10a)
Total Momentum Change Derived from the Universal Force
We know that force is the change of momentum with time (called impulse): F\left(t\right)=\frac{dp}{dt}, dp=F\left(t\right)dt, and finally: \int_{p_1}^{p_2}dp=\int_{t_1}^{t_2}F\left(t\right)dt
The change in momentum (impulse) is then: P=J=\int_{t_1}^{t_2}F\left(t\right)dt=mv_f-mv_i, which we can simply write as:
P=\int_{t_1}^{t_2}F\left(t\right)dt (11)
What time interval should we choose?
Let’s define t_1=0 when the initial velocity is zero (v_i=0), and t_2=\frac{r}{v}, with “r” being the distance between charge centers in the system, and “v” the final relative velocity of the system of charges. Note that the final velocity must be different from zero for the momentum to exist.
Then, the change in momentum can be calculated as follows:
P=\int_{0}^{\frac{r}{v}}F\left(t\right)dt (12)
The force to be integrated is the Universal Electrodynamic Force (valid for any motion), which is given by the following formula:
F_u=\frac{k\cdot q_1\cdot q_2}{r^2}\cdot\frac{\left(\left(1-\beta^2\right)\cdot r+\frac{2\cdot r^2}{c^2}\cdot a\cdot\cos{\left(\alpha\right)}\right)}{r\cdot\left(1-\beta^2\cdot\sin^2{\left(\theta\right)}\right)^\frac{1}{2}}-\frac{k\cdot q_1\cdot q_2}{r^2}\cdot\frac{\left(1-\beta^2\right)\cdot\left(\left(\beta^2\cdot r^2\cdot\cos^2{\left(\theta\right)}+\frac{r^3\cdot a\cdot\cos{\left(\alpha\right)}}{c^2}\right)\cdot r-\beta\cdot r^3\cdot\cos{\left(\theta\right)}\cdot\frac{v\cdot\cos{\left(\theta\right)}}{c}-\frac{r^4}{c^2}\cdot a\cdot\cos{\left(\alpha\right)}\right)}{r^3\cdot\left(1-\beta^2\cdot\sin^2{\left(\theta\right)}\right)^\frac{3}{2}} (13)
Where \beta=\frac{v}{c}, and r,\ v,\ a, are the relative position, velocity, and acceleration between the two charges. In general, velocity and acceleration may not have the same direction. Let’s define their angles with respect to the vector \vec{r}.
\theta: angle between \vec{r} and \vec{v}
\alpha: angle between \vec{r} and \vec{a}
Now let’s integrate the force (13), P=\int_{0}^{\frac{r}{v}}F_udt. The result of this integral gives us the total momentum change for a system of two charges:
P=\frac{1}{\sqrt{1-\sin^2{\left(\theta\right)}\cdot\frac{v^2}{c^2}}}\cdot\left(\frac{kq_1q_2}{rv}+\frac{2kq_1q_2\cos{\left(\alpha\right)}}{vc^2}\cdot a-\frac{kq_1q_2}{rc^2}\cdot v\right) (14)
The total momentum is affected by a factor \gamma_P=\frac{1}{\sqrt{1-\sin^2{\left(\theta\right)}\cdot\frac{v^2}{c^2}}}, which is physically realistic compared to the known “Lorentz factor”, because it also considers the type of motion that is given by the angle \theta between \vec{r} and \vec{v} (the relative position and velocity). Note that the “Lorentz factor” is only valid for circular motion. As mentioned in previous paragraphs, the Theory of Relativity is unable to describe the variables for any type of motion.
We can write the total momentum change for any motion as:
P=\gamma_P\cdot\left(\frac{kq_1q_2}{rv}+\frac{2kq_1q_2\cos{\left(\alpha\right)}}{vc^2}\cdot a-\frac{kq_1q_2}{rc^2}\cdot v\right) (15)
Where 1\le\gamma_P\le\infty, depending on the angle \theta between \vec{r} and \vec{v} and the relative velocity, reaching a maximum value (or \infty) for \theta=\frac{\pi}{2}.
The total momentum in the Theory of Relativity is incomplete. It doesn’t include an acceleration term, as we have derived here from the Universal Electrodynamic Force. As stated before, in the Theory of Relativity, the only kinetic variable is velocity. But you will never know what acceleration was needed to reach that velocity. That’s a basic error in such a theory.
As \gamma_P affects the whole equation, let’s analyze only the terms inside the parentheses to find the meaning of each one of them.
The first term is the equivalent to the torque per unit velocity (\frac{\tau}{v}) in Mechanics. The second term, depending on acceleration, accounts for a momentum component for linear and non-linear motion, which is determined by the angle \alpha between \vec{r} and \vec{a}.
The Universal Electrodynamic Force contains a term that causes the helical motion (corkscrew motion) of charges. This term is responsible for radiation. The acceleration here is the centripetal acceleration, so we can make an equivalent of the angular momentum per velocity squared unit (\frac{L}{v^2}).
The last term proportional to the velocity is perhaps more evident to identify as the classical product that defines linear momentum, i.e., \left(m_{sys}\cdot v\right), with m_{sys}=\frac{kq_1q_2}{rc^2}, being the equivalent mass of the system. Then, we can write the mechanical equivalent of the total momentum change as follows:
P=\gamma_P\left(\frac{\tau}{v}+\frac{L}{v^2}\cdot a-m_{sys}\cdot v\right) \left[\frac{Kgm}{s}\right] or \left[Ns\right]
However, in our system of charges, it makes more sense to define the equation of the total momentum change in terms of the energy related more directly to the charges. The first term is the potential energy or rest energy per unit velocity (\frac{U}{v}=\frac{E_0}{v}=\frac{kq_1q_2}{rv}). The second term, depending on acceleration, is the radiation energy per unit velocity (\frac{E_{rad}}{v}), with E_{rad}=\frac{2kq_1q_2\cos{\left(\alpha\right)}}{c^2}\cdot a. Remember that the Universal Electrodynamic Force contains a term that causes the helical motion (corkscrew motion) of charges and is responsible for radiation.
The last term is like the first, and gives the potential energy or rest energy per velocity squared unit for a constant value of velocity given by “c” (speed of light), multiplied by “v”, (\frac{U}{c^2}\cdot v=\frac{E_0}{c^2}\cdot v=\frac{kq_1q_2}{rc^2}\cdot v).
Putting all this together, we can write the total momentum change of the system as:
P=\gamma_P\left(\frac{E_0}{v}+\frac{E_{rad}}{v}-\frac{E_0}{c^2}\cdot v\right), which, in a more simplified form, becomes:
P=\gamma_P\left(\frac{E_0}{v}\left(1-\frac{v^2}{c^2}\right)+\frac{E_{rad}}{v}\right) (16)
During the development of the present study, we are going to make some calculations of total energy and total momentum change to demonstrate the extent of Universal Electrodynamic Force.
Conclusions
The total energy for a system of charges has been derived from the Universal Force, which seems to be superior to the calculation methods offered in Classical Mechanics and Relativity Theory.
The derived formula for total energy, besides including the rest energy, also has the necessary kinetic variables such as velocity and acceleration. A similar formula is totally absent in Relativity Theory and other theories pretending to calculate the total energy of a system.
It has been demonstrated that there is a relationship between the force and the total energy for zero-force, zero-energy, maximum force, and maximum energy.
It has also been demonstrated that the interaction distance between single charges, nuclei, atoms, or molecules is dynamic and depends on the kinetics of the system. It means that the “Van Der Waals radii” assigned to elements in the periodic table is totally false.
Bibliography
[1]. Raul Fattore, “Negative Mass and Negative Refractive Index in Atom Nuclei – Nuclear Wave Equation – Gravitational and Inertial Control” (2023), Part-1, Part-2, Part-3, Part-4, Part-5, Part-6
[2]. Raul Fattore, “What is Charge? – The Redefinition of Atom – Energy to Matter Conversion” (2023), https://physics-answers.com/what-is-charge-the-redefinition-of-atom-energy-to-matter-conversion/
[3]. David L. Bergman, “Shape & Size of Electron, Proton & Neutron” (2004), Foundations of Science
[4]. N. Graneau, T. Phipps Jr, and D. Roscoe, “An experimental confirmation of longitudinal electrodynamic forces” (2001), The European Physical Journal, https://www.researchgate.net/publication/225353333_An_experimental_confirmation_of_longitudinal_electrodynamic_forces
[5]. V. Milián-Sánchez, F. Scholkmann, et al., “Fluctuations in measured radioactive decay rates inside a modified Faraday cage: Correlations with space weather” (2020), Nature, https://www.nature.com/articles/s41598-020-64497-0
[6]. J.M. Mack, et al., “Remarks on detecting high-energy deuterium–tritium fusion gamma rays using a gas Cherenkov detector” (2006), Los Alamos National Laboratory, Lawrence Livermore National Laboratory, https://www.sciencedirect.com/science/article/abs/pii/S0969806X05003683
[7]. G.R. Mackenzie, et al., “A diamond gammavoltaic cell utilizing surface conductivity and its response to different photon interaction mechanisms” (2021), School of Physics, University of Bristol, https://www.sciencedirect.com/science/article/abs/pii/S2468606921000538
[8]. M. G. Spencer, T. Alam, “High power direct energy conversion by nuclear batteries” (2019), Applied Physics Reviews. 6. 031305. 10.1063/1.5123163, https://www.researchgate.net/publication/335809562_High_power_direct_energy_conversion_by_nuclear_batteries
[9]. K. Hashizume, et al., “Direct Energy Conversion from Gamma Ray to Electricity Using Silicon Semiconductor Cells” (2010), MRS Online Proceeding Library Archive. 1264. 1264-BB05. 10.1557/PROC-1264-BB05-01, https://www.researchgate.net/publication/330650579_Direct_Energy_Conversion_From_Gamma_Ray_to_Electricity_Using_Silicon_Semiconductor_Cells
[10]. Nie Luo, et al., “Proton bremsstrahlung and its radiation effects in fusion reactors” (2010), Department of Nuclear, Plasma and Radiological Engineeing, Univerisity of Illinois, https://www.sciencedirect.com/science/article/abs/pii/S0920379609002440
[11]. INTERNATIONAL ATOMIC ENERGY AGENCY, Fusion Physics, Non-serial Publications, IAEA, Vienna (2012), https://www.iaea.org/publications/8879/fusion-physics
Related articles:
Nuclear Fusion Enhanced by Negative Mass – A Proposed Method and Device – (Part 1)
Nuclear Fusion Enhanced by Negative Mass – A Proposed Method and Device – (Part 3)
Nuclear Fusion Enhanced by Negative Mass – A Proposed Method and Device – (Part 4)
What is Charge? – The Redefinition of Atom – Energy to Matter Conversion
Charge Radiation Derived from the Universal Electrodynamic Force – Proof of the Cherenkov Effect
Faster-Than-Light Travel Feasible with Negative Mass – Superluminal Dynamics
